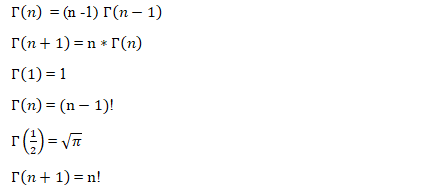The Fascinating Gamma Function in actions

  • Mulatu Lemma Professor
  • Isaac Wright
  • Agegnehu Atena
  • Jonathan Lambright
Keywords: Fascinating Gamma Function in actions

Abstract

The gamma function has several properties that define it. In this paper, I will present proofs for those properties and give example using real numbers. The properties I will prove are as followed:

The_Fascinating_Gamma_Function_in_action

Downloads

Download data is not yet available.

Author Biographies

Mulatu Lemma, Professor

Department of Mathematics

College of Science and Technology

Savannah State University USA

Isaac Wright

Department of Mathematics

Savannah State University

USA

Agegnehu Atena

College of Science and Technology, Department of Mathematics, Savannah State University, USA.

Jonathan Lambright

College of Science and Technology, Department of Mathematics, Savannah State University, USA.

References

M. Abramowitz and I. Stegun, Handbook of Mathematical Functions, Dover, New York, (1964)
G.E. Andrews, R. Askey and R. Roy, Special functions, Cambridge University Press, Cambridge, (1999)
E. Artin, The Gamma Function, New York, Holt, Rinehart and Winston, (1964)
E.W. Barnes, The theory of the gamma function, Messenger Math. (2), (1900), vol. 29, p. 64-128.
P.M Binet, Journal ´ecole polyt., (1839), vol. 16, p. 131
H. Bohr and I. Mollerup, Loerbog I matematisk Analyse, Kopenhagen,
Published
2021-04-30
How to Cite
Lemma, M., Wright, I., Atena, A., & Lambright, J. (2021). The Fascinating Gamma Function in actions. GPH - International Journal of Mathematics, 4(04), 45-54. Retrieved from https://gphjournal.org/index.php/m/article/view/405
Issue
Vol 4 No 04 (2021): GPH-International Journal of Mathematics
Section
Articles

Copyright (c) 2021 GPH - International Journal of Mathematics

Creative Commons License

This work is licensed under a Creative Commons Attribution-NonCommercial-NoDerivatives 4.0 International License.

Author(s) and co-author(s) jointly and severally represent and warrant that the Article is original with the author(s) and does not infringe any copyright or violate any other right of any third parties, and that the Article has not been published elsewhere. Author(s) agree to the terms that the GPH Journal will have the full right to remove the published article on any misconduct found in the published article.